Visitors who see this fresco at the Met museum are often amazed at what seems to be a pre-Renaissance understanding of perspective. One visitor wrote that this “looks like an entire city–perspectivally rendered! The Middle Ages lost those lessons on perspective for sure.”
The statement picks up on a very common triumphalist attitude towards perspective. Perspective is a lesson to be learned by all good art students, it is the golden standard of realism, and the Renaissance Masters either discovered it or rescued it, after the utter ignorance of the Middle Ages.
But what do we mean by “perspective”? Did the Romans use linear perspective? And is the linear model really the best anyone could come up with?
Linear perspective
Linear perspective is the type which was systematized in the Renaissance. It follows several strict rules. One of the important ones is that there can only be one horizon line, and all vanishing points must lie on the same single horizon line.
The above sketch shows an oblong shape rendered in two-point linear perspective. Imaginary lines extend outwards from all horizontal edges. The points where these lines converge are called vanishing points. And of course, all vanishing points are level with the horizon.
A Roman perspective
Now we’ve brushed up on the rules of linear perspective, let’s test and see if the architectural frescoes from the Villa of Publius Fannius Synistor obey these rules. This villa was uncovered at Boscoreale, about one and a half kilometers north of Pompeii.
I’ve selected one of the paintings on the wall of Synistor’s cubiculum. It is a symmetrical painting, but unfortunately the only high resolution photograph of it I could find was framed off-center. This says something about our changing tastes in art, too – we don’t like symmetrical compositions any more. But in any case, the rules of perspective will not be affected by how much of the picture is visible.
At first this painting seems very promising. All the lines of the colonnade in the far background converge quite neatly on one spot in the middle of the painting. It looks like the start of true one-point perspective.
But as the investigation moves to other areas of the painting, things get complicated. The horizontal surfaces at the tops of the foreground columns all converge on a different point – a much higher point – than the columns in the background.
The vanishing point moves again and again, as you include different elements in the picture. But there are two consistent tendencies at work. Firstly, there is symmetry in this composition. Objects to the right and to the left of the centre mirror each other, such that all the vanishing points are aligned along one vertical axis in the middle of the composition. The picture may flagrantly violate linear perspective rules, but the composition is not irrational. Its symmetry shows some level of foresight and planning. It is not something you would arrive at by sheer accident.
Secondly, there seems to be a rule of thumb that the higher elements in the composition have higher vanishing points, and the lower objects have lower vanishing points. This painting, at least, is fairly consistent about where vanishing points ought to be, even though according to the rules of linear perspective they shouldn’t be arranged vertically like this at all.
The vertical bracket here shows how far the imaginary horizon line wanders up and down the picture. The variance amounts to about 40% or more of the height of the picture itself.
Needless to say, this is not linear perspective. It is a different kind of perspective which has different rules. It seems to be more flexible than the Renaissance system. For reasons I will later explain, I will call this type of perspective “eyeball perspective.”
But before we try to systematize this pre-Renaissance perspective theory, let’s look at another symmetrical composition.
This painting was found in the same room, the same cubiculum, as the painting before. It was on a wall facing the other painting. The similarities in style strongly suggest that this was painted by the same artist (or artists) as whoever made the previous picture.
As before, the same kinds of tendencies start to emerge. Objects mirror each other, and their vanishing points are aligned on a vertical axis.
But in this picture there’s an extra variable. Some elements seem to have relatively more parallel-looking lines, while others more obviously seem to be converging. The lines on the floor are slightly more parallel to each other than the shrine walls above.
The benches in the foreground show the greatest degree of parallelism. It almost seems like they do not converge at all towards any vanishing point.
Because of the varying degrees of parallelism, this painting does not obviously seem to follow the rule of thumb outlined above. Objects higher in the composition do not necessarily have higher vanishing points than objects lower in the composition.
And yet, despite all of these variances, these wandering horizons and varying levels of parallelism, the painting doesn’t look too bad. It may not be entirely consistent, it may thumb its nose at the rules of linear perspective, but the effect is not jarring.
And that, actually, is more than what can be said for the perspective drawings which art students today are taught to produce.
It takes a while, and a bit of fiddling around, but it seems no one really notices just how broken the linear perspective system is.
What do you mean, linear perspective is broken?
Well, let’s go back to our example drawing.
This is the oblong shape in two-point perspective. Looking pretty fine so far. But I want to add another oblong shape below it. I’ll take my trusty ruler and extend the lines from those vanishing points below my current shape.
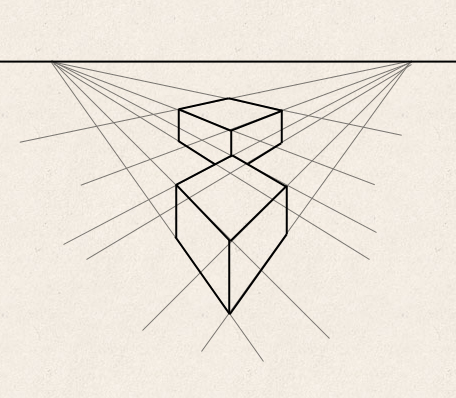
Oh dear. That didn’t turn out well. It looks horrible. I have never seen a right-angled corner that sharp in real life. It’s an impossibility.
Turns out, you can only draw linear perspective as long as everything fits inside a fairly tight window. Outside of this window, it all looks horribly distorted. If you want to fit another oblong shape below this oblong shape, you either have to rub everything out and put your vanishing points further apart, or shrink all of your objects to fit within the window you have.
The Romans did something different. They eyeballed their compositions and moved their horizon lines accordingly. Like this.
Here, the second oblong shape, a smaller boxish shape, has its own imaginary horizon line. Now of course this violates the rules of linear perspective – you can’t have more than one horizon line! That’s inconsistent. But notice how natural it looks. If you didn’t rule up all the lines to see where they met, you wouldn’t necessarily be able to tell that we used a different system of perspective. You would think the same things as that visitor at the Met museum – it looks like perspective. It’s not linear, it’s not mathematically quantified, and it wasn’t invented by the Renaissance. But, goshdammit, it works.
And this is what I can call eyeball perspective. So long as you don’t mix too much parallelism into it, the rules are:
- There are multiple horizon lines.
- Symmetrical elements, or elements aligned the same horizontal distance from the viewer, share horizon lines.
- Elements higher in the composition have higher horizon lines; elements lower down have lower horizon lines.
- Each horizon line can have one or two vanishing points. (This corresponds to one-point and two-point perspective in the linear model and functions the same way)
- Vanishing points are vertically aligned with the corresponding points on different horizon lines.
And this is the style of perspective employed by our Roman fresco painter. It’s not more primitive or naive, nor is it more calculated than the Renaissance model. It is actually more complex, in that it involves several more factors than the linear model. Because of its complexity, the artist has to use his or her eyeball to judge whether the picture looks balanced or not, whether something seems to stick out too much, or where any kind of adjustment needs to be made. It is both rational and organic, naturalistic and formal. It works well enough in both Roman and medieval art.
But why does it work? How could our eyes see something wrong according to the linear model, and interpret it as something right?
Limitations of linear perspective
There is a critical factor which makes the linear perspective model stop working beyond its narrow window.
Eyeballs.
We don’t see the world exactly the way a camera does. Cameras, unless they have special wide-angle-lenses, have a very narrow range of sight. The film takes a static image of whatever it is pointed at, plus a few degrees around it. That’s not how we form a picture of the world. We swivel our eyeballs. We do it constantly, because the best colour vision cells in our retinas are all clustered within a very small zone. So our eyes are constantly flitting around in our sockets. Our brain picks up all of what our eyes rove over, and stitches this together into a big picture of the whole scene.
Now, this would not be too much of a problem for the linear model. As long as all angles of our sight originate from one fixed point, the system should work. But can you see the problem with applying this fixed-point model to the tiny vision-spot of our retinas?
This is how linear perspective assumes our eyeballs see wide angles:
And this is how our eyeballs actually swivel:
As long as our eyeballs stay nested in our sockets, they will rotate on a central axis. This means that points at the extremities of the ball will move around as the ball swivels. Our retinas are at the back extremity of our eyeballs, and only one small spot on them has decent colour vision. That means that when we raise our eyeballs to the heavens, our point-of-vision moves downwards, and when we look down our noses at the ground, our point-of-vision moves upwards.
By the rules of perspective, the horizon line moves when your point-of-vision moves. By insisting that there can only be one horizon line, linear perspective assumes that there is only one point of vision, and it never moves up or down. This is a vast oversimplification of the real situation. As a result, linear perspective has far more in common with the way a camera sees the world than how a human being sees the world, and it cannot accurately represent objects outside of a narrow range of sight.
Conclusions?
Perhaps the question shouldn’t be so much “how could a non-linear system of perspective ever look convincing?”, but “why do we put so much stock in linear perspective?” It is true that the Roman system does not appear to have been quantified mathematically, and so eyeballing involved a fair amount of guesswork and rule-of-thumbing. But that doesn’t mean it was irrational, or that the results were necessarily of lower quality.
The triumph of linear perspective might actually be overstated. In the early 20th century, analytical cubists were seriously dissatisfied with the limitations of linear perspective. They attempted to paint with a much more complex and less predictable system of constantly changing viewpoints. They butchered, sliced and diced up the sacred cow of linear perspective.
Of course, the cubists and the Romans had very different tastes. The cubists were so interested in the question of how to break out of linear perspective, they initially painted in muted colours to better focus on the fragmentation of forms within multiple viewpoints. Romans loved bright colours, and if the writings of Pliny the Elder are any guide, they tended to prefer naturalistic renditions of people and spaces. But what these groups shared was a common disregard for the golden rules of one- and two-point linear perspective. Perhaps art history isn’t such a progressive tale of ever-mounting achievement. Perhaps it’s cyclical after all.
Found in Antiquity 
17 responses to “Romans paint better perspective than Renaissance artists”
Hi, do you have sources for this article? It’s pretty great, and I have never found anything so succinct before. Did you do the illustrations by yourself? Please contact me via my email adress, I’m doing research on optics in art. Thanks!
I don’t know your email address, but I can answer here. The Pompeiian frescoes were from the cubiculum of the Villa of Publius Fannius Synistor. As for factual sources for this article, I spoke from what I learned of perspective and art history in art school. And yes I did do the illustrations myself.
Your art classes are definitely better than ours ^^ Can’t you see my email address? I typed it into the WordPress comment window.
Do you have a contact address somewhere? I don’t want to publish my email address on the web.
Linear perspective does not require all vanishing points to be located on the horizon, only those of objects that are ‘level’ to the ground. If a road is going uphill for instance, its vanishing point moves above the horizon. An image drawn using linear perspective often has many multiple vanishing points for different objects in different orientations.
Of course I’m not suggesting the Roman wall painters were working on this principle – it wouldn’t make any sense for benches to be sloped upwards in relation to the red walls for instance. They were clearly working on a slightly more loose, eyeball system as you suggest, a kind of not-quite-fully-systematised-linear-perspective.
Ok…linear perspective isn’t broken. Sometimes it’s implemented in a fashion that doesn’t necessarily yield ‘natural results’. Not indicative of a broken system, just indicative of a poorly-implemented system. In the sample used in the above article the primary issue is that the author has used too narrow a field of view vs. that of a human eye. Our vanishing points can be simulated accurately – they just end up ‘off the page’. Just because it’s not convenient for us doesn’t mean it’s not true.
(There’s a comment on this page about height of horizons. We do only have one, at a given eye-level. In that, the artical is correct.)
Yes there is only one horizon, which corresponds to our eye level. This article is incorrect though, in saying that linear perspective requires all vanishing points to be located on that horizon line. As I said above, that only applies to objects that are ‘level’ on the ground.
My apologies for returning to this late – I bumped into the page bookmark and noticed your reply.
What you refer to (with respect to hills) may APPEAR to be a “different” vanishing point, heading off to a non-eye-level horizon, but its not…not really.
In reality, if we consider a road heading up a constant gradient hill directly in front of us (we are at the bottom), then the sides of the road would appear to converge at a vanishing point off the horizon. The truth of the matter is that things are still converging in accordance with the single ‘viewer’s eye level’ horizon rule (which is just physics).
It’s tricky to describe without an associated sketch, but if we drew a neat 3D box around the road using only vertical and horizontal lines, and put that box into the image, we’d see the top of the box (which is co-planar with the road on ‘top’ of the hill) converges to our eye-level horizon. This would also be true of the sides of the box (the sides would be co-planar with the sides of the road). The road is essentially a diagonal plane within the box, hence the illusion it goes to a different vanishing point.
Mathematically, physically and in reality, each viewer really does only have one horizon, and that really is level with their point of view. All other perceived notions are falacies.
(3D animation can look completely realistic. They use the Cartesian co-ordinate system (x,y,z) and universal truths w.r.t. perspectives to mathematically render a scene. A correctly hand-drawn image always uses exactly the same principles, but we model the principles graphically rather than figuring it out numerically…and are slower 🙂
Yes, as I have said, there is only one horizon / eye-line, that much is fact – our eyes can only be in one place at once.
In your reply, however, you have basically demonstrated that I was correct. Anything level to the ground appears to have a vanishing point located on the horizon – including your imaginary box and the level bit of road “on top of the hill”. All these things are co planar. The surface of the inclined road, however, is a separate plane, arranged diagonally within the box, as you have said. It is not co-planar and is pointing upwards (from our position). Therefore the sides of the road appear to converge at a vanishing point located above the horizon.
An inclined plane has a vanishing point above the horizon, a declined plane has one located below the horizon. Simple.
Please look these images and tell me that they’re incorrect? That the vanishing points are all really on the horizon?
Note in the first example that all the ‘flat’ sections of road converge on the horizon, whilst the declined section has a vanishing point well below.
You say it is an illusion – well, every vanishing point is an illusion. It is the consequence of translating 3d reality onto a 2d plane. That process is the creation of illusion – the illusion of depth on a flat surface.
Perhaps this is why you are confusing linear perspective in drawing with linear perspective as applied to 3d graphics? After all the version of a street as rendered in a computer program is still in three dimensions, albeit in virtual space. Whereas a drawing is an illusionistic suggestion of three dimensions whilst only actually existing in two.
I’m not sure if the ‘rules’ of linear perspective apply in the same way to 3d design (I have no expertise in CAD) but I definitely know how they apply in drawing (which is the subject of this article).
Hi Gordon,
Yes, there is only one horizon/eye-line, this much is fact – we can only have one set of eyes.
In your comment, however, you have actually demonstrated that I am correct in my initial statement (vanishing points are not necessarily located on the horizon).
In your example, the flat ground which we stand on, the flat part “at the top of the hill” and the top of the imaginary box are all co-planar. Therefore they all have a vanishing point located on the horizon. The surface of the road that is inclined, however, is a separate plane, arranged diagonally in the imaginary box. This plane is pointing upwards (from where we’re standing) and therefore its sides appear to converge at a point located above the horizon.
An inclined plane has a vanishing point above the horizon, a declined plane has one below the horizon. Simple.
Please look at these images and tell me that they’re wrong? That the vanishing points are all actually on the horizon line?
Note in the first example, that the flat sections of road converge on the horizon, whilst the declined section has a vanishing point well below.
You say that it is merely an illusion.
Well, all vanishing points are an illusion – the very process of making a perspectival drawing is the creation of an illusion. It is the illusion of depth created on a flat surface.
Making an illusionistic drawing is nothing to do with what’s really there (we know that roads don’t actually get more narrow, and walls don’t get smaller the further they are from us – those lines are always parallel) and everything to do with perception. A perspective drawing is like a sheet of glass between us and the scene; a flat surface on which we can ‘fix’ our perceptions of depth.
Perhaps this is the root of a confusion between perspective as applied to drawing and in 3d design? After all, a digitally modelled version of a street is still existing in three-dimensions, albeit existing in virtual space. A drawing however, is the translation of three-dimensions onto a 2d plane, rather a different activity.
I don’t know whether perspective applies differently to 3d design (I have no expertise in CAD) but I know how it applies practically in making a drawing.
Apologies for posting twice, I thought my browser has screwed up so wrote it all again!
Brilliant. I felt that something strange was going on, looking up at some things and down at others, but I did not associate it with eye-balling at the Pompei exhibition. I have felt it in the horizontal plane when looking at haystacks by Monet. I am not sure how it works in the horizontal plane but it felt as if he were looking more than one direction and this made the haystacks come alive, or the scene come alive. You should turn this blog article into a journal article.
I wonder if my comment was rejected or i failed to post it. I think your theory is brilliant and should be published in a more formal format.
I think that Monet also used a similar technique in some of his landscapes except in the horizontal plane depicting that which he could see by moving his eyeballs. This means for instance that one could see more of the haystacks that one could see from a single camera perspective. I attempted to make a horizontal eyeball image from two halves of a stereoscop image since in the horizontal plane, not only do we move our eyeballs but we have two eyeballs so our right eye is providing more information on the right side, and our left eye is providing more information regarding our left side visual field. If recreated on a two dimensional image, it would be an “eyeballs perspective.”
https://www.flickr.com/photos/nihonbunka/33504397085/in/photostream/
A very consice insightful essay. As a student of art most of my life I find your essay most intriguing!
Hi Carla….yes a very interesting subject but the article does require some further consideration…. The Roman perspectives you consider are not 2 point perspective attempts whereas the demonstration you draw is using 2 point perspective…( you would be hard up to find a 2 point perspective in the renaissance let alone roman times.!)using your analysis and your comment that Romans ‘prefer naturalistic representations of people and spaces’…I would gather that these Roman artists would greatly have enjoyed the benefits of the linear system developed in the Renaissance…as there is no doubt that although there are distortions with the system when the field of view is extended as you point out…on the whole it serves very well to represent the illusion of space…As someone who has studied both architecture and fine art and worked as a manual perspective artist I can confirm that Michael Chance is correct about inclined planes..when we draw a ramp or roof etc on an incline we use a vanishing point above the horizon line or below the horizon line depending on whether the plane is ascending or descending….hope this helps to clarify the very interesting issues you raise…
[…] 2013. Romans Paint Better Perspective Than Renaissance Artists [Online]. Available: https://foundinantiquity.com/2013/08/09/pompeiian-fresco-painters-used-perspective-better-than-renai… [Accessed 20 August […]
I’ve enjoyed reading “Romans Paint Better Perspective Than Renaissance Artists”,
posted on the 9th of August 2013 by Carla Hurt. It’s sad that we have none of the mathematical treatises on Perspective cited in ancient texts, and only a very few surviving examples of any Greek or Roman paintings. With so little ancient evidence to study, it feels impossible to form conclusions of certainty.
Though the Renaissance Linear Perspective system today continues to proliferate (especially in its photographic form); there are other 20th century Perspective systems whose structures might better pertain to whatever theories underlay the ancient Greek and Roman pictures. The first is the ongoing development of illustration of wide-angle views, most frequently seen as the “Curvilinear Perspective” popularized by Flocon and Barre (1968, translated by artist Robert Hansen in 1987). Matching more closely with the evidence of ancient Italian mural paintings is the idea of illustrations using multiple points-of-view systematized by Kevin Forseth in his 1984 book “Glide Projection”. His system(s) seems (to me) to give a better hint at the geometrical rationalization of what Erwin Panofsky called “the roving eye” of the Roman muralists.
My own 2 page essay on this Greco-Roman Perspective (2011) is at: http://7ladders.com/Essays.htm
The overall conclusion of Carla Hurt’s post is quite important – perspective is today in a period of re-thinking and new development – it is a living, still-growing, set of ideas –not a finalized solution pertaining only to its Italian Renaissance form.
Jim Barnes, Oklahoma City – 27th September 2020